원함수 는 여기로 연결됩니다. 어떤 함수를 도함수로 하는 함수에 대해서는
부정적분 문서를 참고하십시오.
코사인 은 여기로 연결됩니다. 아프리카 남부의 민족에 대해서는
코사족 문서를 참고하십시오.
사인 함수와 코사인 함수 수학 에서 삼각 함수 (三角函數, 영어 : trigonometric functions, angle functions, circular functions 또는 goniometric functions )는 각 의 크기를 삼각비 로 나타내는 함수 이다. 즉, 삼각형의 각도와 변의 길이의 관계를 나타낸 것이다. 예각 삼각 함수는 직각 삼각형 의 예각 에 직각 삼각형의 두 변의 길이의 비를 대응시킨다. 임의의 각의 삼각 함수 역시 정의할 수 있다. 삼각 함수는 복소수의 지수 함수 의 실수 · 허수 부분이며, 따라서 복소수 를 다룰 때 핵심적인 역할을 한다. 가장 근본적인 주기 함수 이며, 각종 주기적 현상을 다룰 때 푸리에 급수 의 형태로 등장한다.
삼각 함수에는 3개의 기본적인 함수가 있으며, 이들은 사인 (영어 : sine , 문화어 :
sin
{\displaystyle \sin }
코사인 (영어 : cosine , 문화어 :
cos
{\displaystyle \cos }
탄젠트 (영어 : tangent , 문화어 :
tan
{\displaystyle \tan }
코시컨트 (영어 : cosecant , 기호
csc
{\displaystyle \csc }
시컨트 (영어 : secant , 기호
sec
{\displaystyle \sec }
코탄젠트 (영어 : cotangent , 기호
cot
{\displaystyle \cot }
직각 삼각형 C가 직각인 삼각형 ABC에서, 각 A, B, C의 대변(마주보는 변)의 길이를
a
,
b
,
h
{\displaystyle a,b,h}
사인 , 코사인 , 탄젠트 의 정의는 다음과 같다.
사인:
sin
A
=
a
h
{\displaystyle \sin A={\frac {a}{h}}}
코사인:
cos
A
=
b
h
{\displaystyle \cos A={\frac {b}{h}}}
탄젠트:
tan
A
=
a
b
{\displaystyle \tan A={\frac {a}{b}}}
또한, 코시컨트 , 시컨트 , 코탄젠트 는 위 세 함수의 역수가 되며, 다음과 같이 정의한다.
코시컨트:
csc
A
=
h
a
=
1
sin
A
{\displaystyle \csc A={\frac {h}{a}}={\frac {1}{\sin A}}}
시컨트:
sec
A
=
h
b
=
1
cos
A
{\displaystyle \sec A={\frac {h}{b}}={\frac {1}{\cos A}}}
코탄젠트:
cot
A
=
b
a
=
1
tan
A
{\displaystyle \cot A={\frac {b}{a}}={\frac {1}{\tan A}}}
삼각 함수 좌표평면 에서 원점을 중심으로 하고 반지름 r의 길이가 1인 원을 단위원 이라고 한다. 이 단위원 위의 점 A
(
x
,
y
)
{\displaystyle (x,y)}
x
{\displaystyle x}
θ
{\displaystyle \theta }
sin
θ
=
y
r
{\displaystyle \sin \theta ={\frac {y}{r}}}
cos
θ
=
x
r
{\displaystyle \cos \theta ={\frac {x}{r}}}
tan
θ
=
sin
θ
cos
θ
=
y
x
{\displaystyle \tan \theta ={\frac {\sin \theta }{\cos \theta }}={\frac {y}{x}}}
sec
θ
=
1
cos
θ
{\displaystyle \sec \theta ={\frac {1}{\cos \theta }}}
csc
θ
=
1
sin
θ
{\displaystyle \csc \theta ={\frac {1}{\sin \theta }}}
cot
θ
=
1
tan
θ
=
cos
θ
sin
θ
{\displaystyle \cot \theta ={\frac {1}{\tan \theta }}={\frac {\cos \theta }{\sin \theta }}}
오일러의 공식
e
i
x
=
cos
x
+
i
sin
x
{\displaystyle \,e^{ix}=\cos x+i\sin x}
x
=
b
i
{\displaystyle \,x=bi}
e
−
b
=
cos
b
i
+
i
sin
b
i
{\displaystyle \,e^{-b}=\cos bi+i\sin bi}
x
=
−
b
i
{\displaystyle \,x=-bi}
e
b
=
cos
(
−
b
i
)
+
i
sin
(
−
b
i
)
=
cos
b
i
−
i
sin
b
i
{\displaystyle \,e^{b}=\cos(-bi)+i\sin(-bi)=\cos bi-i\sin bi}
연립하여 풀면, 쌍곡선함수 ,
cos
b
i
=
e
b
+
e
−
b
2
=
cosh
b
{\displaystyle \cos bi={\frac {e^{b}+e^{-b}}{2}}=\cosh b}
i
sin
b
i
=
−
e
b
+
e
−
b
2
,
{\displaystyle i\sin bi={{-e^{b}+e^{-b}} \over 2}\;,}
−
i
sin
b
i
=
e
b
−
e
−
b
2
=
sinh
b
{\displaystyle -i\sin bi={{e^{b}-e^{-b}} \over 2}=\sinh b}
사인 · 코사인 · 코시컨트 · 시컨트는 주기가
2
π
{\displaystyle 2\pi }
주기함수 이다. 즉, 임의의 복소수
z
∈
C
{\displaystyle z\in \mathbb {C} }
sin
z
=
sin
(
z
+
2
π
)
{\displaystyle \sin z=\sin(z+2\pi )}
csc
z
=
csc
(
z
+
2
π
)
{\displaystyle \csc z=\csc(z+2\pi )}
sec
z
=
sec
(
z
+
2
π
)
{\displaystyle \sec z=\sec(z+2\pi )}
탄젠트 · 코탄젠트는 주기가
π
{\displaystyle \pi }
주기함수 이다. 즉, 임의의 복소수
z
∈
C
{\displaystyle z\in \mathbb {C} }
tan
z
=
tan
(
z
+
π
)
{\displaystyle \tan z=\tan(z+\pi )}
cot
z
=
cot
(
z
+
π
)
{\displaystyle \cot z=\cot(z+\pi )}
사인 과 코사인 은 실수선 위에서 해석함수 이며, 복소 평면 위에서 정칙함수 이다. 이들은 복소 무한대
∞
^
{\displaystyle {\hat {\infty }}}
본질적 특이점 을 갖는다.[ 1] [ 2]
탄젠트는 실수선의
π
/
2
+
n
π
{\displaystyle \pi /2+n\pi }
n
∈
Z
{\displaystyle n\in \mathbb {Z} }
사인과 코사인의 그래프
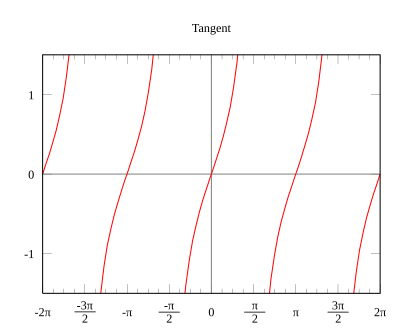
탄젠트 그래프
코시컨트 그래프
단위원 위의 각 점의 좌표 특별한 각 에서의 삼각 함수의 값은 다음과 같다.
180
∘
=
π
r
a
d
{\displaystyle {180^{\circ }}={\pi }\;\mathrm {rad} }
라디안 )
특수각
sin
cos
tan
0
{\displaystyle 0}
0
{\displaystyle 0}
1
{\displaystyle 1}
0
{\displaystyle 0}
π
/
6
{\displaystyle \pi /6}
1
/
2
{\displaystyle 1/2}
3
/
2
{\displaystyle {\sqrt {3}}/2}
1
/
3
{\displaystyle 1/{\sqrt {3}}}
π
/
4
{\displaystyle \pi /4}
2
/
2
{\displaystyle {\sqrt {2}}/2}
2
/
2
{\displaystyle {\sqrt {2}}/2}
1
{\displaystyle 1}
π
/
3
{\displaystyle \pi /3}
3
/
2
{\displaystyle {\sqrt {3}}/2}
1
/
2
{\displaystyle 1/2}
3
{\displaystyle {\sqrt {3}}}
π
/
2
{\displaystyle \pi /2}
1
{\displaystyle 1}
0
{\displaystyle 0}
정의되지 않음
0º , 90º sin, cos, tan 각 사분면에 따른 삼각 함수의 부호는 다음과 같다.
사분면
sin과 csc
cos과 sec
tan와 cot
I
+
+
+
II
+
−
−
III
−
−
+
IV
−
+
−
삼각 함수 사이에는 많은 항등식이 존재한다. 그중 가장 자주 쓰이는 것은 피타고라스 항등식 으로, 어떤 각에 대해서도 사인의 제곱과 코사인의 제곱의 합은 1이다. 이는 반지름의 길이가
r
{\displaystyle r}
b
,
{\displaystyle b,}
x
{\displaystyle x}
a
{\displaystyle a}
a
2
+
b
2
r
2
=
r
2
r
2
=
1
{\displaystyle {\frac {a^{2}+b^{2}}{r^{2}}}={\frac {r^{2}}{r^{2}}}=1}
피타고라스의 정리 로 설명할 수 있다. 이를 삼각 함수로 나타내면 다음과 같다.
sin
2
x
+
cos
2
x
=
1
{\displaystyle \,\sin ^{2}x+\cos ^{2}x=1}
이것은 다음과 같다.
sin
2
x
+
cos
2
x
=
1
{\displaystyle \sin ^{2}x+\cos ^{2}x=1}
(
a
r
)
2
+
(
b
r
)
2
=
1
{\displaystyle \left({a \over r}\right)^{2}+\left({b \over r}\right)^{2}=1}
(
a
2
r
2
)
+
(
b
2
r
2
)
=
1
{\displaystyle \left({a^{2} \over r^{2}}\right)+\left({b^{2} \over r^{2}}\right)=1}
a
2
+
b
2
r
2
=
r
2
r
2
=
1
{\displaystyle {a^{2}+b^{2} \over r^{2}}={r^{2} \over r^{2}}=1}
a
2
+
b
2
=
r
2
=
1
∵
r
=
1
{\displaystyle a^{2}+b^{2}=r^{2}=1\;\because \;r=1}
따라서, 이것은 또한 단위원 에서 다음과 같다.
(
3
2
)
2
+
(
1
2
)
2
=
1
{\displaystyle \left({{\sqrt {3}} \over 2}\right)^{2}+\left({1 \over 2}\right)^{2}=1}
서로 다른 삼각 함수의 관계는 삼각 함수의 덧셈 정리 제2 코사인 법칙 과 두 점 사이의 거리 공식 을 연립해 유도할 수 있고, 제1 코사인 법칙 과 사인 법칙 을 연립해 유도할 수 있고, 오일러의 공식 을 이용해 유도할 수도 있다.
sin
(
x
±
y
)
=
sin
x
cos
y
±
cos
x
sin
y
,
{\displaystyle \sin \left(x\pm y\right)=\sin x\cos y\pm \cos x\sin y,}
cos
(
x
±
y
)
=
cos
x
cos
y
∓
sin
x
sin
y
{\displaystyle \cos \left(x\pm y\right)=\cos x\cos y\mp \sin x\sin y}
두 각의 크기가 같을 경우에는 덧셈정리를 간단하게 배각공식을 이용할 수 있다.
모든 삼각 함수는 다른 삼각 함수를 사용하여 다음과 같이 나타낼 수 있다.
sin
cos
tan
cot
sec
csc
sin
sin
x
{\displaystyle \sin x}
1
−
cos
2
x
{\displaystyle {\sqrt {1-\cos ^{2}x}}}
(
tan
x
)
/
1
+
tan
2
x
{\displaystyle (\tan x)/{\sqrt {1+\tan ^{2}x}}}
1
/
cot
2
x
+
1
{\displaystyle 1/{\sqrt {\cot ^{2}x+1}}}
sec
2
(
x
)
−
1
/
(
sec
x
)
{\displaystyle {\sqrt {\sec ^{2}(x)-1}}/(\sec x)}
1
/
(
csc
x
)
{\displaystyle 1/(\csc x)}
cos
1
−
sin
2
x
{\displaystyle {\sqrt {1-\sin ^{2}x}}}
cos
x
{\displaystyle \cos x}
1
/
1
+
tan
2
(
x
)
{\displaystyle 1/{\sqrt {1+\tan ^{2}(x)}}}
(
cot
x
)
/
cot
2
x
+
1
{\displaystyle (\cot x)/{\sqrt {\cot ^{2}x+1}}}
1
/
(
sec
x
)
{\displaystyle 1/(\sec x)}
csc
2
x
−
1
/
(
csc
x
)
{\displaystyle {\sqrt {\csc ^{2}x-1}}/(\csc x)}
tan
(
sin
x
)
/
1
−
sin
2
x
{\displaystyle (\sin x)/{\sqrt {1-\sin ^{2}x}}}
1
−
cos
2
x
/
(
cos
x
)
{\displaystyle {\sqrt {1-\cos ^{2}x}}/(\cos x)}
tan
x
{\displaystyle \tan x}
1
/
(
cot
x
)
{\displaystyle 1/(\cot x)}
sec
2
x
−
1
{\displaystyle {\sqrt {\sec ^{2}x-1}}}
1
/
csc
2
x
−
1
{\displaystyle 1/{\sqrt {\csc ^{2}x-1}}}
cot
1
−
sin
2
x
/
(
sin
x
)
{\displaystyle {\sqrt {1-\sin ^{2}x}}/(\sin x)}
(
cos
x
)
/
1
−
cos
2
x
{\displaystyle (\cos x)/{\sqrt {1-\cos ^{2}x}}}
1
/
(
tan
x
)
{\displaystyle 1/(\tan x)}
cot
(
x
)
{\displaystyle \cot(x)}
1
/
sec
2
x
−
1
{\displaystyle 1/{\sqrt {\sec ^{2}x-1}}}
csc
2
x
−
1
{\displaystyle {\sqrt {\csc ^{2}x-1}}}
sec
1
/
1
−
sin
2
x
{\displaystyle 1/{\sqrt {1-\sin ^{2}x}}}
1
/
(
cos
x
)
{\displaystyle 1/(\cos x)}
1
+
tan
2
x
{\displaystyle {\sqrt {1+\tan ^{2}x}}}
cot
2
x
+
1
/
(
cot
x
)
{\displaystyle {\sqrt {\cot ^{2}x+1}}/(\cot x)}
sec
x
{\displaystyle \sec x}
(
csc
x
)
/
csc
2
(
x
)
−
1
{\displaystyle (\csc x)/{\sqrt {\csc ^{2}(x)-1}}}
csc
1
/
(
sin
x
)
{\displaystyle 1/(\sin x)}
1
/
1
−
cos
2
x
{\displaystyle 1/{\sqrt {1-\cos ^{2}x}}}
1
+
tan
2
x
/
(
tan
x
)
{\displaystyle {\sqrt {1+\tan ^{2}x}}/(\tan x)}
cot
2
x
+
1
{\displaystyle {\sqrt {\cot ^{2}x+1}}}
(
sec
x
)
/
sec
2
x
−
1
{\displaystyle (\sec x)/{\sqrt {\sec ^{2}x-1}}}
csc
x
{\displaystyle \csc x}
다음은 6개의 기본 삼각 함수에 대한 도함수와 부정적분이다.
함수
f
(
x
)
{\displaystyle f(x)}
도함수
f
′
(
x
)
{\displaystyle f'(x)}
부정적분
∫
f
(
x
)
d
x
{\displaystyle \textstyle \int f(x)\,dx}
sin
x
{\displaystyle \sin x}
cos
x
{\displaystyle \cos x}
−
cos
x
+
C
{\displaystyle -\cos x+C}
cos
x
{\displaystyle \cos x}
−
sin
x
{\displaystyle -\sin x}
sin
x
+
C
{\displaystyle \sin x+C}
tan
x
{\displaystyle \tan x}
sec
2
x
{\displaystyle \sec ^{2}x}
−
ln
|
cos
x
|
+
C
{\displaystyle -\ln \left|\cos x\right|+C}
cot
x
{\displaystyle \cot x}
−
csc
2
x
{\displaystyle -\csc ^{2}x}
ln
|
sin
x
|
+
C
{\displaystyle \ln \left|\sin x\right|+C}
sec
x
{\displaystyle \sec x}
sec
x
tan
x
{\displaystyle \sec {x}\tan {x}}
ln
|
sec
x
+
tan
x
|
+
C
{\displaystyle \ln \left|\sec x+\tan x\right|+C}
csc
x
{\displaystyle \csc x}
−
csc
x
cot
x
{\displaystyle -\csc {x}\cot {x}}
ln
|
csc
x
−
cot
x
|
+
C
{\displaystyle \ln \left|\csc x-\cot x\right|+C}
사인 법칙 은 임의의 삼각형 ABC에서 각 A , B , C 의 대변 a , b , c 에 대해 다음과 같은 관계를 만족함을 나타낸다.
sin
A
a
=
sin
B
b
=
sin
C
c
{\displaystyle {\frac {\sin A}{a}}={\frac {\sin B}{b}}={\frac {\sin C}{c}}}
마찬가지로,
a
sin
A
=
b
sin
B
=
c
sin
C
=
2
R
{\displaystyle {\frac {a}{\sin A}}={\frac {b}{\sin B}}={\frac {c}{\sin C}}=2R}
도 성립한다. 여기서 R 은 삼각형의 외접원의 반지름의 길이를 나타낸다.
코사인 법칙 에는 총 두 가지의 법칙이 있다.
코사인 제 1 법칙 에 따르면,
c
=
b
cos
A
+
a
cos
B
{\displaystyle c=b\cos A+a\cos B}
양변의 길이와 알고자 하는 변 사이의 두 각의 크기를 알 경우, 다른 한 변의 길이를 알아낼 때 사용할 수 있다.
코사인 제 2 법칙 은 피타고라스의 정리 를 확장한 것이다.
c
2
=
a
2
+
b
2
−
2
a
b
cos
C
{\displaystyle c^{2}=a^{2}+b^{2}-2ab\cos C}
가 성립하고, 위의 식을 변형하면
cos
C
=
a
2
+
b
2
−
c
2
2
a
b
{\displaystyle \cos C={\frac {a^{2}+b^{2}-c^{2}}{2ab}}}
와 같이 나타낼 수 있다.
코사인법칙은 두 변의 길이와 끼인각의 크기를 알 때 삼각형의 나머지 한 변의 길이를 구할 때 유용하게 쓸 수 있다. 또한 모든 변의 길이를 알고 있을 때 각의 코사인값을 구할 때에도 사용할 수 있다.
탄젠트법칙은 임의의 삼각형 ABC에서 각 A , B 의 대변 a , b 에 다음과 같은 식을 만족시킨다.
a
+
b
a
−
b
=
tan
1
2
(
A
+
B
)
tan
1
2
(
A
−
B
)
{\displaystyle {\frac {a+b}{a-b}}={\frac {\tan {{1 \over 2}(A+B)}}{\tan {{1 \over 2}(A-B)}}}}
기원전 2~1세기 그리스의 히파르코스 와 프톨레마이오스 등은 각도에 대해 달라지는 현 의 길이를 다룬 적이 있다.
현재 쓰는 것과 같은 삼각 함수의 원형은 굽타 시대 인도 천문학에서 찾아볼 수 있다. 기원후 4~5세기 인도의 천문학 책이 산스크리트어에서 아랍어를 통해 라틴어로 번역되면서 유럽에 전해졌다. 5세기 초 발간된 인도 의 천문학 서적 『수우르야 싯단타(Sūrya Siddhānt, 태양에 관한 지식)』에는 세계 최초로 삼각 함수에 관해 정확하고 자세하게 표현된 설명이 기록되어 있다.[ 3]
삼각 함수가 동아시아에 전해진 것은 16~17세기 때이다.
영어 ‘사인(sine )’은 라틴어 sinus 에서 왔는데, 이는 12세기의 유럽 번역가들이 아랍어 جَيْب (jayb )를 ‘옷의 목부분, 옷깃’으로 보고 라틴어로 번역한 것이다. 하지만 이 단어는 실제로는 ‘활시위 ’를 뜻하는 산스크리트어 ज्या (jyā , 베다 jiyā́ )를 음차한 것이다.
‘탄젠트(tangent )’는 ‘접한다’는 뜻의 라틴어 tangens 에서 왔고, ‘시컨트(secant )’는 ‘자른다’는 뜻의 라틴어 secans 에서 왔다. 각각 원에 접하는 선과 자르는 선에 빗대어 붙인 이름이다.
코사인, 코탄젠트, 코시컨트의 ‘코(co-)’가 처음 쓰인 책으로는 에드먼드 건터 (영어판 ) Canon triangulorum (1620년)이 있는데, ‘여각 의 사인’(sinus complementi )을 ‘코사인(cosinus )’으로 줄여 부른 것이다.
한자 문화권 에서는 독일의 선교사·과학자인 요한 슈렉 (영어판 ) 정현 (正弦)·여현 (餘弦)·정절 (正切)이라고 번역했다. 코탄젠트·시컨트·코시컨트는 각각 여절 (餘切)·정할 (正割)·여할 (餘割)이라 한다. 이 이름은 근대화되기 전의 조선·일본에서 쓰였고, 지금도 중국에서 쓰인다.