큰 이십면체

큰 이십면체
종류	케플러-푸앵소 다면체
별모양화 중심	정이십면체
원소	F = 20, E = 30 V = 12 (χ = 2)
면의 수{변의 수}	20{3}
슐레플리 기호	{3,5/2}
면 배치	V(53)/2
위토프 기호	5/2 | 2 3
콕서터 다이어그램
대칭군	Ih, H3, [5,3], (*532)
참조	U53, C69, W41
특성	정다면체 비볼록 델타다면체
(35)/2 (꼭짓점 도형)	큰 별모양 십이면체 (쌍대다면체)

기하학에서 큰 이십면체(great icosahedron)는 슐레플리 기호가 {3,5/2}고 콕서터 다이어그램이 이며 케플러-푸앵소 다면체(비볼록 정다면체) 네 개 중 하나디이다. 이것은 교차하는 삼각형 면 20개가 꼭짓점에서 오각성의 순서로 다섯 개가 만나게 이루어져 있다.

그림

투명한 모형	밀도	별모양화 도표	전개도
큰 이십면체의 투명한 모형(애니메이션을 보라)	이 절단면에서 보였듯이 밀도가 7이다.	이것은 정이십면체의 별모양화이고, 웨닝거 모델 [W41]이며 정이십면체의 17가지 별모양화 중 16번째이고 콕서터의 59가지 별모양화 중 7번째이다.	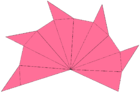× 12 전개도; 이등변삼각형으로 이루어진 별 오각뿔 열두 개를 정십이면체의 면처럼 배열한다. 각 각뿔은 선풍기 날개처럼 접힌다: 점선은 다면체 선과는 반대 방향으로 접는다.

구면 타일링

이 다면체는 밀도가 7인 구면 타일링을 나타낸다. (윤곽선을 파란색으로, 내부를 노란색으로 칠한 구면 삼각형 하나를 위에서 나타냈다)

다듬은 다면체로

큰 이십면체는 다른 색을 칠한 면과 유일하게 정사면체 대칭만을 가지는 다듬은 고른 다면체로 구성된다: . 이 구성은 깎은 정팔면체(또는 부풀려 깎은 정사면체)의 부분적인 면으로 인해서 정이십면체의 다듬은 정사면체 대칭과 유사하게 역다듬은 정사면체또는 역다듬은 사사면체라고 불린다: . 이것은 또한 삼각형을 2가지로 색칠할 수 있고 황철면체 대칭 또는 으로 구성될 수 있고, 역다듬은 정팔면체라고 부른다.

정사면체 대칭	황철면체 대칭

관련 다면체

이것은 볼록 정이십면체와 동일한 꼭짓점 배열을 가진다. 이것은 또한 작은 별모양 십이면체와 동일한 모서리 배열을 가진다.

큰 이십면체에 반복적으로 적용되는 깎기 연산은 고른 다면체의 수열을 만든다. 깎아서 모서리를 점으로 만들면 절반 깎은 큰 이십면체로 큰 이십십이면체를 만들어 낸다. 이 과정은 원래의 면을 점으로 만드는 완전 깎기가 될 때까지 계속되며 큰 별모양 십이면체를 만들어낸다.

깎은 큰 별모양 십이면체는 깎아낸 꼭짓점에서 삼각형 면 20개와 원래 오각성 면을 깎아서 나온 두배가 된 (숨겨진)오각형 면({10/2})이 12개로 정이십면체와 모서리를 공유하면서 포함되는 큰 십이면체를 두 개 만드는 불가능한 다면체이다.

이름	큰 별모양 십이면체	깎은 큰 별모양 십이면체	큰 십이이십면체	깎은 큰 이십면체	큰 이십면체
콕서터 다이어그램
그림

참고 문헌

• Wenninger, Magnus (1974). 《Polyhedron Models》. Cambridge University Press. ISBN 0-521-09859-9. 
• Coxeter, Harold Scott MacDonald; Du Val, P.; Flather, H. T.; Petrie, J. F. (1999). 《The fifty-nine icosahedra》 3판. Tarquin. ISBN 978-1-899618-32-3. MR 676126.  (1st Edn University of Toronto (1938))
• H.S.M. 콕서터, Regular Polytopes, (3rd edition, 1973), Dover edition, ISBN 0-486-61480-8, 3.6 6.2 Stellating the Platonic solids, pp. 96–104

외부 링크

• Weisstein, Eric Wolfgang. Great icosahedron (Uniform polyhedron). 《Wolfram MathWorld》 (영어). Wolfram Research.
  • Weisstein, Eric Wolfgang. “Fifteen stellations of the icosahedron”. 《Wolfram MathWorld》 (영어). Wolfram Research. 
• Uniform polyhedra and duals

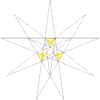
정이십면체의 유명한 별모양화
정다면체	고른 쌍대			정다면체 복합체			별 정다면체	기타
(볼록) 정이십면체	작은 삼각육변형 이십면체	중간 삼각육변형 이십면체	큰 삼각육변형 이십면체	정팔면체 5-복합체	정사면체 5-복합체	정사면체 10-복합체	큰 십이면체	파낸 십이면체	최종 별모양화
정이십면체의 별모양화 과정은 많은 정이십면체 대칭을 가지는 관련 다면체와 복합체를 만든다.