에어리 함수의 그래프 수학 에서 에어리 함수 (Airy function )는 특수 함수 의 한 종류다. 두 개가 있으며, 기호는 Ai와 Bi다. 조지 비델 에어리 가 광학을 연구하기 위해 1838년에 도입하였다.[ 1]
다음과 같은
f
(
x
)
{\displaystyle f(x)}
상미분 방정식 을 에어리 미분 방정식 (Airy differential equation )이라고 한다.
f
″
(
x
)
=
x
f
(
x
)
{\displaystyle f''(x)=xf(x)}
에어리 함수 는 에어리 미분 방정식의 두 개의 독립적인 해로, 에어리 미분 방정식의 일반적인 해는 두 에어리 함수의 선형결합 이다. 에어리 함수는 다음과 같이 적분으로 표현할 수 있다.
Ai
(
x
)
=
1
π
∫
0
∞
cos
(
t
3
/
3
+
x
t
)
d
t
{\displaystyle \operatorname {Ai} (x)={\frac {1}{\pi }}\int _{0}^{\infty }\cos(t^{3}/3+xt)\,dt}
Bi
(
x
)
=
1
π
∫
0
∞
[
exp
(
−
1
3
t
3
+
x
t
)
+
sin
(
1
3
t
3
+
x
t
)
]
d
t
{\displaystyle \operatorname {Bi} (x)={\frac {1}{\pi }}\int _{0}^{\infty }\left[\exp \left(-{\tfrac {1}{3}}t^{3}+xt\right)+\sin \left({\tfrac {1}{3}}t^{3}+xt\right)\right]\,dt}
x
≫
1
{\displaystyle x\gg 1}
A
i
(
x
)
≈
exp
(
−
2
3
x
3
/
2
)
2
π
x
1
/
4
{\displaystyle Ai(x)\approx {\frac {\exp(-{\frac {2}{3}}x^{3/2})}{2{\sqrt {\pi }}x^{1/4}}}}
B
i
(
x
)
≈
exp
(
2
3
x
3
/
2
)
π
x
1
/
4
{\displaystyle Bi(x)\approx {\frac {\exp({\frac {2}{3}}x^{3/2})}{{\sqrt {\pi }}x^{1/4}}}}
A
i
(
−
x
)
≈
sin
(
2
3
x
3
/
2
+
π
/
4
)
π
x
1
/
4
{\displaystyle Ai(-x)\approx {\frac {\sin({\frac {2}{3}}x^{3/2}+\pi /4)}{{\sqrt {\pi }}x^{1/4}}}}
B
i
(
−
x
)
≈
cos
(
2
3
x
3
/
2
+
π
/
4
)
π
x
1
/
4
{\displaystyle Bi(-x)\approx {\frac {\cos({\frac {2}{3}}x^{3/2}+\pi /4)}{{\sqrt {\pi }}x^{1/4}}}}
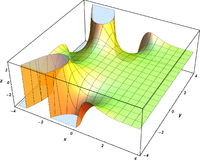
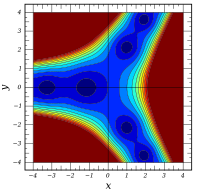
ℜ
[
A
i
(
x
+
i
y
)
]
{\displaystyle \Re \left[\mathrm {Ai} (x+iy)\right]}
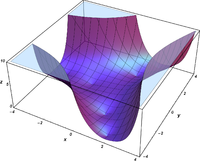
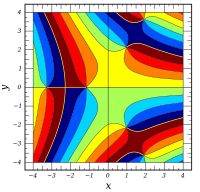
ℑ
[
A
i
(
x
+
i
y
)
]
{\displaystyle \Im \left[\mathrm {Ai} (x+iy)\right]}
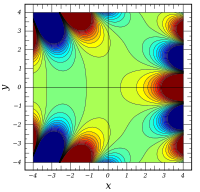
|
A
i
(
x
+
i
y
)
|
{\displaystyle |\mathrm {Ai} (x+iy)|\,}
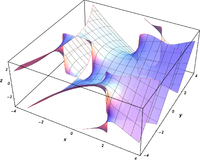
a
r
g
[
A
i
(
x
+
i
y
)
]
{\displaystyle \mathrm {arg} \left[\mathrm {Ai} (x+iy)\right]\,}
ℜ
[
B
i
(
x
+
i
y
)
]
{\displaystyle \Re \left[\mathrm {Bi} (x+iy)\right]}
ℑ
[
B
i
(
x
+
i
y
)
]
{\displaystyle \Im \left[\mathrm {Bi} (x+iy)\right]}
|
B
i
(
x
+
i
y
)
|
{\displaystyle |\mathrm {Bi} (x+iy)|\,}
a
r
g
[
B
i
(
x
+
i
y
)
]
{\displaystyle \mathrm {arg} \left[\mathrm {Bi} (x+iy)\right]\,}
↑ Airy, George Biddell (1838). “On the intensity of light in the neighbourhood of a caustic ”. 《Transactions of the Cambridge Philosophical Society》 6 : 379–402.
Olver, Frank W.J. (1997). 《Asymptotics and Special Functions》 2판. A. K. Peters/CRC Press. ISBN 1568810695 Press, WH; Teukolsky, SA; Vetterling, WT; Flannery, BP (2007), 〈Section 6.6.3. Airy Functions〉 , 《Numerical Recipes: The Art of Scientific Computing》 3판, New York: Cambridge University Press, ISBN 978-0-521-88068-8 원본 문서 에서 보존된 문서, 2012년 9월 12일에 확인함 Vallée, Olivier; Soares, Manuel (2004), 《Airy functions and applications to physics》 , London: Imperial College Press, ISBN 978-1-86094-478-9 MR 2114198 , 2010년 1월 13일에 원본 문서 에서 보존된 문서, 2012년 9월 12일에 확인함 Weisstein, Eric Wolfgang. “Airy Functions” . 《Wolfram MathWorld 》 (영어). Wolfram Research. Wolfram Functions Site: Ai , Bi .















![{\displaystyle \operatorname {Bi} (x)={\frac {1}{\pi }}\int _{0}^{\infty }\left[\exp \left(-{\tfrac {1}{3}}t^{3}+xt\right)+\sin \left({\tfrac {1}{3}}t^{3}+xt\right)\right]\,dt}](https://wikimedia.org/api/rest_v1/media/math/render/svg/399df89c262ba0b1afc6cb814015af2b045f7c17)





![{\displaystyle \Re \left[\mathrm {Ai} (x+iy)\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/505e2f06e2e8d14027c46f1f4b1ac72367f85b58)
![{\displaystyle \Im \left[\mathrm {Ai} (x+iy)\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6c4ca8fdfe9c79b62f9becbb2687b12f68d42e18)

![{\displaystyle \mathrm {arg} \left[\mathrm {Ai} (x+iy)\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/190234ee42ad7ac3a352d501c46e3bfcb4e64be4)
![{\displaystyle \Re \left[\mathrm {Bi} (x+iy)\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3a86d49867d1f711cbe25936ea7982c44f005c53)
![{\displaystyle \Im \left[\mathrm {Bi} (x+iy)\right]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b658626fb2e88ae1d2a3ff37af457b29b0f17e0d)

![{\displaystyle \mathrm {arg} \left[\mathrm {Bi} (x+iy)\right]\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1e6398901714ff29a82ca26b13f90f473377a731)